Measuring attenuation in a fiber-optic cable is a vital ingredient to obtaining the maximum performance from a system designs. But, for designers, just starting to work in the fiber-optic design space, measuring attenuation can seem like a monumental task.
In this tutorial, we’ll take a look at the basics behind attenuation as well as at the impact Maxwell’s equations and a power detector have on attenuation measurements. We’ll then examine the two simple measurement techniques that designers can employ during to ease the attenuation measurement process.
Attenuation Basics
Light propagates in a glass tube because of reflection at the cylinder’s surface by the angle at which the optimal path is totally internal. But light moves in alternating electric and magnetic components at 90 degrees to each other that can be construed as a block waveguide over time.
Attenuation is tested more than predicted. It has been categorized according to some common experiences, and this should be a warning that you are quite capable of coming up with different predictions based on different experience levels.
In the manufacturing process, crystallization of the glass out of a melt results in minor “kinks” in the final product due to impurities. OH water molecules called “high water” are another deformity incorporated into the material during the manufacture that results in absorption. Differences also occur between the surface and the bulk material out of the melt.
Composition, temperature, and pressure are interrelated variables in the manufacturing process, and they all affect the transition from melt to solid. The transition itself is a stage process in which crystallization changes these variables of composition, temperature, and pressure throughout the transition. Inconsistency is the result and inconsistency will cause imperfections resulting in scattering and absorption.
To avoid interpretation differences, designers can use loss measurements to evaluate attenuation on a cable. The calculation of loss is established as a ratio between power launched and power output measured in watts. The decibel conversion is:
Loss(db)=-10log10(Pout/Pin)
Because attenuation features are incorporated in every fiber, they also increase their effect with the length of that fiber. Macrobending, for example, happens in a gradual curve of the tube with the accumulation of kinks; a kind-of systems-effect in distance because the wave propagation takes place over time.
The attenuation number on most data sheets is the quantity of dB/km, where:
A(dB/km)=loss(dB)/fiber length(km)
Other complicating variables occur from environmental conditions in humidity, temperature and vibration. Loose or tight-buffered construction distinguishes indoor from outdoor use, but these environmental factors are also important in a laboratory when trying to measure at the most sensitive levels.
Maxwell’s Equations
Wave propagation is understood by Maxwell’s equations describing the field effects. Field effects explain how the fiber core is penetrated, and inform the use of the cladding, but they are generally important for the entire discipline of fiber-optic technology. The wave equations have an equivalent relationship with each other, and translate by integral as well as second-order partial differential equations. These equations explain the nature of electromagnetic (EM) waves propagating in a lossy medium, and the propagation in waveguides as well as resonance.
Maxwell’s four equations describe wave propagation. The first equation  represents Gauss’s law that bounds the charge by the enclosed surface and relates to the total refractive index.
represents Gauss’s law that bounds the charge by the enclosed surface and relates to the total refractive index.
Maxwell’s second equation  represents Faraday’s law of electromagnetic induction where the electromotive force recommends fiber optic bit rate and bandwidth compared with purely electrical conduction.
represents Faraday’s law of electromagnetic induction where the electromotive force recommends fiber optic bit rate and bandwidth compared with purely electrical conduction.
The third equation  describes the relationship of electric and magnetic field coordinates that extends the wave propagation outside the core, and the fourth equation
describes the relationship of electric and magnetic field coordinates that extends the wave propagation outside the core, and the fourth equation  relates to the universal constant of vacuum permeability μ0.
relates to the universal constant of vacuum permeability μ0.
In general, these equations let us know how the fields of an EM wave relate to one another, as well as propagate in a medium. Light travels as an ideal, unbounded dielectric where both vectors, electric and magnetic, are perpendicular to the direction of propagation (the z axis) according to the second and third laws.
Fiber limitations in conductivity, permitivity, and permeability of the medium relate to features of the first and fourth law with the result that an electromagnetic field propagating in a medium takes the form of damping waves. This has an attenuation constant also known as the propagation constant because of the dependent frequency effects in the conducting fiber. EM radiation in the high frequency of light is perfectly conducted, and yet glass is only a perfect diaelectric for a low-frequency alternating current. The result is attenuation.
In addition to attenuation, the solutions for Maxwell’s equations yield another feature important in fiber-optic cable technology: discrete, eigenvalue waveguides. Waveguides describe stable field patterns that are one of the advantages of digital transmission in stability with modal features compared to analog signals. These are important for the design and fabrication of multi-mode fibers, but it relates here as background to indicate why the unique EM wave properties are an advantage in fiber optic technology. With single-mode fibers, the phase properties that are displayed as modes are less important than the polarization features of light. Thus, a polarization mode dispersion (PMD) value heads most single mode fiber data sheets.
There are several tricks to compensate for attenuation, and we want to conclude here that attenuation is to-be-expected. Our question was how much attenuation is taking place in the cable, but the complicating system includes the laser source and the power meter.
Determining Bit Rate
Bit rate is one area that attenuation has a big impact in. The bit rate that can be transmitted, measured in bit/s, relates to the bandwidth, measured in Hertz, that is the frequency range within which a signal can be transmitted without significant deterioration. Bit rate and bandwidth (BW) are often related as BW = BR/2because one period of sine wave requires two bits of characterization information.
A fiber-optic provides between 100 and 1000 THz in light frequency compared to radio frequencies of 500 kHz to 100 MHz, coaxial cable up to 100 MHz, and copper wire, over a short distance, up to 1 MHz.
Multiplexing
Maximizing the bit rate transmission are modulation schemes that take advantage of the wave nature in combination with the refractive control. Control distinguishes one carrier from another, and the wave features allow several configurations each of which can be beneficially overlapped, one with the other.
Sonet features carrier switched data in frames transmitting in intervals of 51.84 Mbit/s. As many as 48 of these OC-n levels are possible for a total of 48 * 51.84 = 2.488 Gbits. Groups of OC-ns are called “superframes.”
Communication companies use multiplexing to increase the bandwidth by improving these devices at the ends without having to install new fiber. With the right type of fiber, however, you can, in principle, have a device that does both multiplexing and demultiplexing.
The nature of the signal informs the varying ways that multiplexing can be accomplished. Consider signals coded in close sequence with time division multiplexing (TDM), or carrying signals at different frequencies in wave division multiplexing (WDM). Add these together and you can achieve multiple waves at different times with dense wave division multiplexing (DWDM).
These complex multiplexing combinations use statistical methods to keep track of the overlapping patterns. The nature of the signal interacts between theory, device, and mathematics resulting in a rich communication channel.
The Detector
Power detection is a vital element for determining the exact attenuation on a fiber-optic cable. A typical, medium-grade detector is a power meter that registers 0.001-dB typical polarization dependent response with a single input port for both connectors and bare fiber measurements (Figure 1).
 Figure 1: Illustration of a typical power detector used for fiber-optic cable testing.
Figure 1: Illustration of a typical power detector used for fiber-optic cable testing.
Fiber connector adapters are usually included with the power detector along with a changeover configuration from bare fiber measurements. These connectors can be threaded, or simply slipped into the adapter hopefully comparable with a bare fiber where the adapter locates the fiber ferrule at the same place in the integrating cavity as the bare fiber. The angle of the cleave, possibly magnified by the connection, reflections, and absorptions will introduce measurement noise just as they do between the source and the fiber.
The power meter measures light by turning it into electrical current where an efficiency rating called the responsivity is measured in units of amperes per watt (A/W). A photodiode typically converts optical power to current, but thermal detectors are also used because sometimes a measure independent of the wavelength is important. The source problems are duplicated in the detector with efficiency calibration of the photodiode because the responsivity is a function of wavelength.
Thermal detectors, on the other hand, directly convert optical to electrical power like a large sink into which all the splashing water can be measured as it is caught in the basin. It won’t tell you about the flow of power, however, and a photodiode is more sensitive in that regard. Photodiodes are capable of measuring power levels down to less than 1 pW (-90 dBm). The thermal plates, in contrast, produce a proportional measure where linearity is irrelevant because the only aim is to achieve equal temperature between two layers.
The highest performance solution is to use a tunable laser as the source and an optical spectrum analyzer (OSA) to cover a broad wavelength range. An OSA provides additional filtering to reject some of the broadband noise emission from tunable lasers, and the combination provides a large measurement range and fine wavelength resolution in most measurements.
In this article, lets consider a bootstrap method that can be done as an initial test of two attenuation measures for insertion loss apart from the electronics of bandwidth broadcast and power metering. One of the reasons for this simpler approach is that OSA’s can be expensive, but another is that automated detectors only solve part of the measurement problem.
The craft of working with fiber optic cable dominates the management of source, connectors, and meter with temperature stabilization, power range non-linearity, polarization, reflection, interference, angled fiber cleaves, and other, systematic certainties and uncertainties. Variations on spectrum analysis, for example, can be devised using interferometry techniques just as variations in measurement can be devised simply with configurations of circulators and isolators.
Simple tests are possible because fiber-optic expertise is a craft that automation has not overcome. As long as the source and the detector consistently operate in the same way over some assuring time period, we can perform two simple insertion loss experiments for measuring attenuation.
Simple Attenuation Measurements
As mentioned in the paragraph above, two simple insertion loss experiments can be used to measure for attenuation (Figure 2). If we assume consistency at the ends, we can use an attenuation effect to measure two quantities just within the cable. Light is attenuated in the ratio dB/km, and we can measure the difference between two configurations; one where the length or bending is minimal, compared (measured) against one where the length or bending is extensive.
 Figure 2: Designers can measure attenuation differences using the two methods show here.
Figure 2: Designers can measure attenuation differences using the two methods show here.
The beauty of this measurement is that it does not depend on the lack of control inherent in any system. Reasonable stability is the only requirement, and we can reasonably measure that over some reassuring time period.
The most important step is simply confirming that you have a signal. Designers might be delayed at this point while you develop skill in the cleaving process.
With a signal in place, time measures will help designers become comfortable with the system’s stability. Wiggle the fiber, jump up and down on the floor, and open the windows to vary the environmental factors.
Designers can change features of the system like the length of the fiber as well as changing and recording environmental conditions. In our laboratory, our experimentalist bought several lengths of fiber in graduations of feet to test a more sophisticated measure of coherence length for reflection concerns. But the principle is the same that even sophisticated answers can be tested in simple configuration tests.
Calculating the principle in mathematics provides a numerical reference of this analysis. Will you get a graduated measure of attenuation due to length because of the dispersion features in the wave propagation and the manufacturing defects in the cable gradually accumulating over distance? Defining this one feature of attenuation with the attendant control problems will not be unlike defining the most sophisticated fiber optic attenuation questions. Testing the limits of attenuation in length is a simple approach that nevertheless involves many complicating features of working with fiber-optic cable. This playful part of experimentation further entangles designers with the system as they get more comfortable swapping modules in and out and watching the results.
Attenuation from bending can be done in the same way. Wrapping the fiber around cylinders of different diameters will logically result in degradations of the signal due to attenuation. Snell’s index of internal reflection will cease at some coiled limit, the attenuation might gradually increase as the coil tightens, or the cable will simply break.
Designers looking to use the methods described above should see if they can obtain the three following results: no effect, attenuation matched to diameter, and broken coil. Number one and three shouldn’t be too hard. But, designers must grapple with understanding at what point does attenuation turn into an interrupted signal?
Designers should also try to achieve result number two to reassure themselves that a null result isn’t poor experimentation, and to justify the money spent on broken coil.
Wrap Up
These two simple measures are a beginner’s way to get familiar with the measurement of signals in a fiber optic cable by independently making two measures of attenuation. Attenuation results from an interesting combination of theory, devices, and configuration where unravelling the signal features will depend on craftsmanship. The system can become increasingly complex with just a few components, and this beginning will lay a foundation for understanding, building, and trouble-shooting more extensive networks. In any system, we commonly find a source, a transmission line, and the receiver. Connecting and testing these in a fiber optic cable system is one approach to understanding the nature of measurement which is the nature of error.

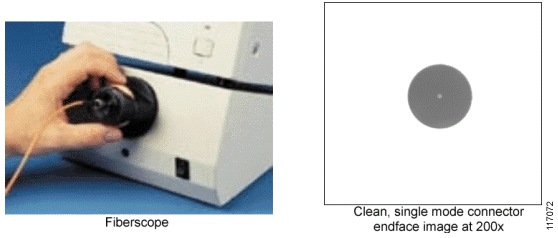
 Caution: Read the reminders and warnings before you begin this process.
Caution: Read the reminders and warnings before you begin this process. Warning: Invisible laser radiation might be emitted from disconnected fibers or connectors. Do not stare into beams or view directly with optical instruments.
Warning: Invisible laser radiation might be emitted from disconnected fibers or connectors. Do not stare into beams or view directly with optical instruments.